To help you understand just how this happens, assume we have two investors with the opportunity to invest in either STRIPS or a five-year bond. The investor who opts for the 5-year bond which utilize forward rates will have a simple task: they will invest at the onset and then wait to receive regular scheduled coupon payments, plus the principal amount at maturity.
An Econometric Model of the Term Structure of Interest-Rate Swap Yields on JSTOR
The investor who opts for STRIPS which utilize spot rates will roll them over as they mature throughout the 5-year period Rolling over implies that when one STRIP expires, the investor will use the proceeds to invest in the next six-month contract, and so on for 5 years. In market conditions where short-term rates are above the forward rates utilized by bond prices, the investor who rolls over the STRIPS will tend to outperform the investor in the 5-year bond.
The opposite is true: In market conditions where short-term rates are below the forward rates utilized by bond prices, the investors who rolls over the STRIPS will tend to underperform the investor in the 5-year bond. The most and widely accepted yield curves pit the three-month versus two-year T-bonds, or the five-year versus ten-year T-bonds.
On occasion, the Federal Funds Rate versus the year Treasury note may be used. The yield curve typically slopes upwards indicating that the rate of interest on long-term bonds is higher than the rate on short-term bonds. Such a curve is said to be normal. Other than a normal yield curve, we could have a flattening yield curve or a steepening yield curve. A flat yield curve indicates that little if any, difference exists between short-term and long-term rates for similarly rated bonds. It may manifest as a result of long-term interest rates falling more than short-term interest rates or short-term rates increasing more than long-term rates.
Copy Citation
A flattening curve reflects expectations of investors about the macroeconomic outlook. It may be the result of the following events:. A trader who anticipates a flattening of the yield curve can buy a long-term rate and sell short a short term rate because they expect bond prices to rise in the long-term. A steepening yield curve indicates a widening gap between the yields on short-term bonds and long-term bonds.
A steepening curve could occur when long-term rates rise faster than short-term rates. Sometimes, short-term rates can also show some defiance by decreasing even as long-term rates rise.
Post navigation
By 30 th August, the two-year note could have risen to 2. The difference would effectively go from 1 percentage point to 1.
A steepening curve reflects expectations of investors about the macroeconomic outlook. A trader who anticipates a steepening of the yield curve can sell short a long-term rate and buy a short-term rate because they expect bond prices to fall in the long-term bond prices fall as rates increase. The 6-month forward rate on an investment that matures in 1. Consider a bond with par value of USD 1, and maturity in four years.
- accutane drug coupons!
- Subscribe to RSS.
- Your Answer.
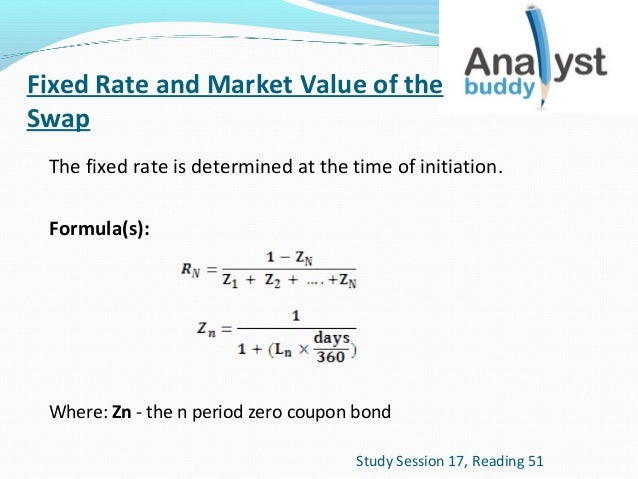
The spot rate curve is as follows:. The value of the bond is the present value of all future cash flows coupons plus principal , discounted at the various spot rates. You must be logged in to post a comment. Calculate discount factors given interest rate swap rates. Compute spot rates given discount factors.
- Interest rate swap - Definition, valuation.
- vegas coupons january 2020!
- columbia outlet store printable coupons!
- Interest Rate Yield Curve Construction and Bootstrapping | FinPricing.
- Zero coupon swap - Wikipedia.
- Zero Coupon Swap.
- Pricing fixed income securities against an interest swap curve.
Interpret the forward rate, and compute forward rates given spot rates. Define par rate and describe the equation for the par rate of a bond. First of all, one needs to have valuation models for each types of instruments. Given a Future price, the yield or zero rate can be directly calculated as. There are two keys in yield curve construction: interpolation and optimization for root finding. Most popular interpolation algorithms in curve bootstrapping are linear, log-linear and cubic spline.
They can be applied to either zero rates or discount factors. Some critics argue that some of those simple interpolations cannot generate smooth forward rates and the others may be able to produce smooth forward rates but fail to match the market quotes. Also they cannot guarantee the continuity and positivity of forward rates. Although the monotone convex interpolation rule sounds almost perfectly, it is not very popular with market practitioners. As described above, the bootstrapping process needs to solve a yield using a root finding algorithm. In other words, it needs an optimization solution to match the prices of curve-generated instruments to their market quotes.
FinPricing employs the Levenberg-Marquardt algorithm for root finding, which is very common in curve construction. Another popular algorithm is the Excel Solver, especially in Excel application.
An Econometric Model of the Term Structure of Interest-Rate Swap Yields
Consequently, market practitioners started to use a new valuation approach referred to as multicurve approach, which is characterized by a unique discounting curve and multiple forecasting curves The current methodology in capital markets for marking to market securities and derivatives is to estimate and discount future cash flows using rates derived from the appropriate term structure.
Yield Curve Construction and Bootstrapping Overview Yield curves are derived or bootstrapped from observed market instruments that represent the most liquid and dominant interest rate products for certain time horizons. Assuming that we have had all yields up to 4 years and now need to derive up to 5 years.
Let x be the yield at 5 years. Use an interpolation method to get yields at 4. Given the 5 year market swap rate, we can use a root-finding algorithm to solve the x that makes the value of the 5 year inception swap equal to zero. Now we obtain all yields or equivalent discount factors up to 5 years Repeat the above procedure till the longest swap maturity. Interpolation Most popular interpolation algorithms in curve bootstrapping are linear, log-linear and cubic spline.
The monotone convex interpolation is more rigorous.